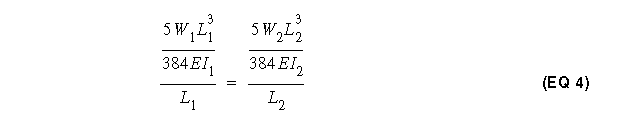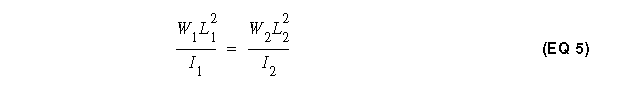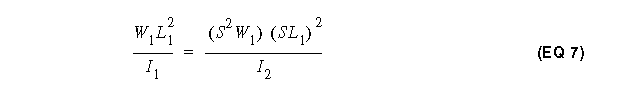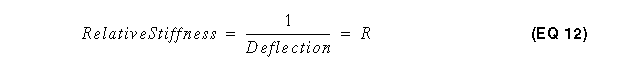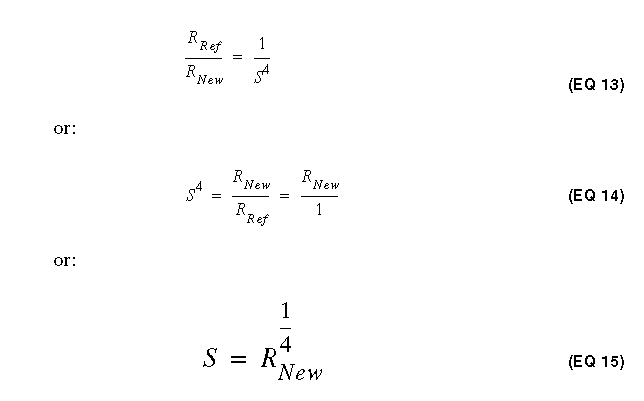Dave Lord's Scale Factor

Dave Lord's scale factor describes the relation of spars' relative stiffness when a kite design is scaled.

A derivation of the formula.

After the publication of my article"Mass-stab" in Drachen Magazin (2/93) and English version of it in rec.kites archive ("Bending spars with a Coke can"), some kite designers have contacted me and asked: "Why the relative stiffness is proportional to the FOURTH power of the scale factor?" The following derivation is based on correspondence with Dave Lord. The derivation logic of the formula is from Dave, my contribution was just to make the layout. I have not written a "scientific" paper for twenty years, please forgive me my exercise quality. In 1995 I converted the document into HTML-format.

The frame of a kite is made of separate spars or beams. In the following we have a look at a loaded beam, supported at two points.

According to the handbook Maximum deflection is:

Where

E = modulus of material

and

I = moment of inertia.

If we make (our kite larger/smaller = ) the beam longer/shorter by a factor of S :

but want (the kite behave similarly) then the relative deflection should be the same:

Substituting (EQ1) into (EQ3):

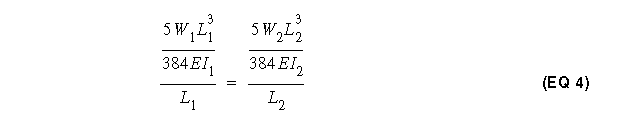
which simplifies to:

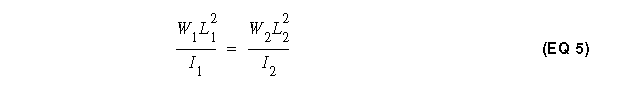
The area of a kite changes by the square of scale (S) and the load (W) is proportional to the area:

substituting (EQ2) and (EQ6) into (EQ5):

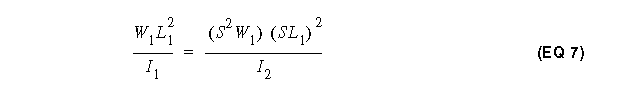
which simplifies to:

Let's define:

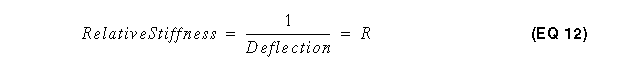
For practical purposes we select a reference spar (e.g. K-75), which has the relative stiffness Rref = 1. Other spar types are compared to it and their stiffness is denoted by Rnew.

From (EQ11) and (EQ12):

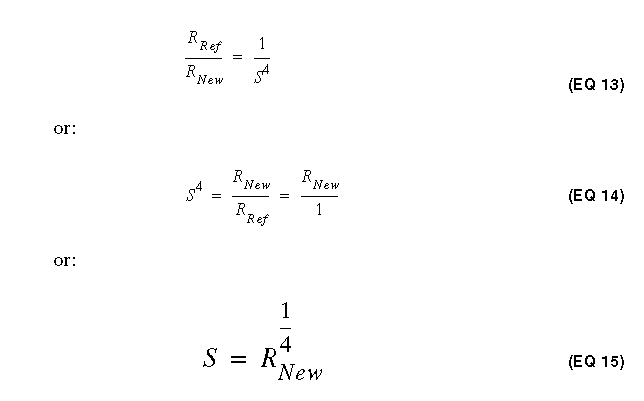
Smooth Winds & Scaling.

Simo.Salanne@csc.fi